Table of contents
Introduction
Volatility is a part of the stock market. It is the term used to gauge how much the price of an asset changes over time. The higher the volatility, the greater the uncertainty and risk associated with the asset. Usually, in the derivative market, the higher the volatility, the better the chance of making a profit.
Volatility is categorised into two types: historical and implied. Historical volatility informs you of how the particular asset has shown fluctuation in its price movement in the past. In contrast, the latter relates to the market forecast. Let’s discuss the implied volatility in options in detail.
What is implied volatility?
Implied volatility (IV) represents what the market anticipates regarding the future volatility of an underlying security in options trading. It is derived from an option contract’s current market price and other factors, including the expiry date, strike price, interest rate, and dividend yield.
Implied volatility tells you how much the market expects a contract to fluctuate or move until the expiry date. As a trader, you may use it to assess the level of risk that exists in the current market. It also helps in selecting which options trading strategy can help you maximise your gains.
Often people get confused between implied volatility and standard deviation as both are related to risk assessment. While they are related but not identical. Let us help you.
Standard deviation measures how much an asset’s price deviates from its mean over a given period. On the other hand, implied volatility is derived from the option prices, which reflect the market’s expectations of future price movements. Standard deviation relies on historical data, reflecting past price movements.
Now that the basics are clear, let’s move on to the next part.
Factors affecting implied volatility
Implied volatility is not a constant value but rather a dynamic one. It changes with the market conditions and the following factors mentioned below.
Demand and supply
When there is more demand for options than supply, the option price and implied volatility increase. Let’s take an example of this.
There is a lot of uncertainty or speculation in the market when a listed company announces its earnings, launches a product, or a major news event comes out. In such a scenario, traders buy options to hedge or profit from the potential price movements. This increases the demand and price of options having that stock as an underlying asset and the result is increased volatility.
Time to expiration
The lengthier the time to expiration, the higher the option price and implied volatility, and vice versa. This happens because when the contract expiration day is far, the underlying asset gets enough time to move in favour of or against the option holder.
Market sentiment
When the market is bullish or optimistic about the future performance of an underlying asset, the option price and the implied value of that call option increase, and the option price and implied volatility of puts decrease, and vice versa.
This is because when the market is bullish, more traders buy calls to profit from the anticipated price increase of the underlying asset and sell puts to reduce the cost of buying the underlying asset. This increases the demand and price of calls and decreases the demand and price of puts.
How to calculate implied volatility in options trading?
There is no direct implied volatility formula. You can compute implied volatility using the Black-Scholes model. This model was developed by Black and Myron Scholes in 1973. It is based on the assumption that the underlying security’s price follows a random and continuous process called a geometric Brownian motion.
That means the price changes are normally distributed and independent of each other and that the expected return and volatility of the asset are constant over time.
The formula for this method for a European call option (that you can exercise only at maturity) is:
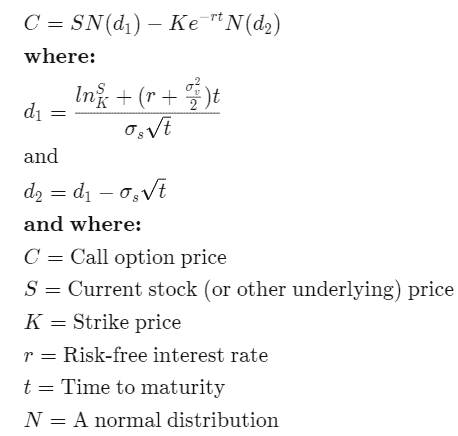
As you can see, the Black-Scholes formula requires you to know the implied volatility of the option, which is not directly observable in the market.
So how do you find it? The answer is to use a trial-and-error method called iteration.
Iteration means starting with an initial guess of the implied volatility and plugging it into the Black-Scholes formula to get the option’s theoretical value. Then, you compare this value with the option’s actual market price, also known as the option premium.
If the theoretical value exceeds the market price, your implied volatility guess is too high, and you need to lower it. If the theoretical value is lower than the market price, your implied volatility guess is too low, and you need to raise it.
Repeat this process until you find the implied volatility that makes the theoretical value and the market price as close as possible. You can also use available IV calculators to simplify this process. You can compute futures implied volatility in the same manner.
How to use implied volatility in options?
By implementing any of the following strategies, you can understand how to use implied volatility in options and implement the same.
Long Straddle
This is a neutral strategy. Here, you buy a put and call option with an identical strike price and expiry. When an underlying asset’s price movement is significant in either direction, you can benefit by executing one contract and letting go of the other one, which expires worthless.
Short Straddle
This works opposite to the long straddle and is ideal when the implied volatility is initially high but is now expected to show a downtrend. Here, you sell a put and a call option with a similar strike price and expiry. If an underlying price does not move much in either direction, you earn from the decrease in the price of the derivative contract.
Iron Condor
To implement this strategy, you buy a call and a put option with a similar strike price and sell a call and put with a higher and a lower strike price, respectively. Here, the options you sold and those you bought must have the same expiration date.
Note that these are only three of yeh widely used strategies, and there are numerous more strategies that you can use.
Conclusion
Implied volatility is a key factor affecting an option’s price and profitability. It is the market’s expectation of the future volatility of an underlying asset. Using this factor, you can identify overpriced or underpriced options and select the optimal strategy for your trading goals and risk appetite.
However, there are other factors to consider in options trading. Also, weigh the historical volatility and the fundamental and technical analysis of the underlying asset. To learn more about such interesting concepts, subscribe to StockGrow blogs!
FAQs
Implied volatility is a market metric that indicates how much movement can be expected in a security’s price over a period of time. It is estimated by various factors, such as market conditions and the data available about the asset.
Low implied volatility indicates that the price fluctuation will be minimal over a span of one year. This is also a plausible time to buy options contracts and enter the markets.
There is no direct formula to compute the implied volatility; however, the Black Scholes Merton Model is often used to find out the implied volatility. There are two formulas: one is for calls, and the other for puts.
Next, iterations are carried out with different implied volatility values, and the value at which the calculated option price is approximately equal to the market options price is taken as the implied volatility.
Your first step is to first determine whether the implied volatility for a particular security is high or low. When the implied volatility is high, identify options premium selling opportunities; if you find option premiums with low implied volatility, it is a potential buying opportunity.
Implied volatility is not a 100% reliable indicator because it does not consider the intrinsic or fundamental value of financial security. However, traders often use this metric, among others, to estimate the magnitude of the price fluctuation and make financial decisions based on the values obtained. However, it is best to use a set of metrics to determine whether an investment should be made.
